What is the protonation state?

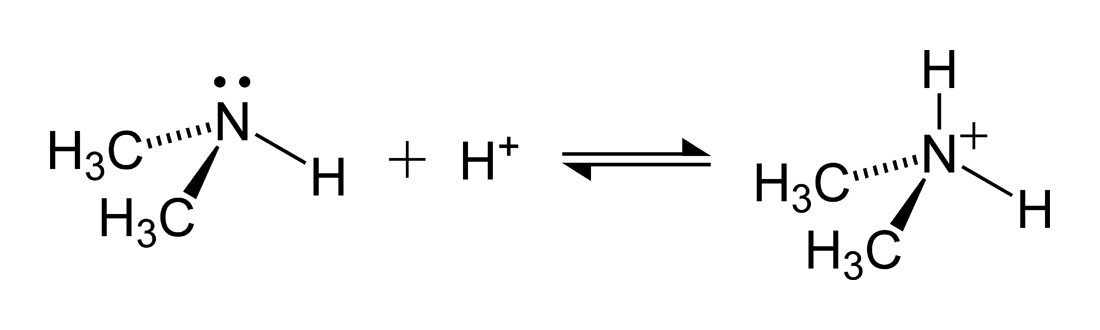
A key idea to consider with any acid/base conjugate pair is the "protonation state" at a given pH.

That is, if you find the molecule in solution at a particular pH, is the proton "on" the molecule (in the protonated form) or "off" the molecule (in the deprotonated form)?

For example, let's consider ammonia

Ammonia, NH₃ is a base and its conjugate acid is the ammonium ion, NH₄⁺. These are essentially two forms of the same compound. One that is deprotonated, the ammonia. One that is protonated, the ammonium. If this compound is found in solution at a particular pH it is important to know which concentration will be larger. This can be deduced by examining the equilibrium constant. As both the protonated form and deprotonated form are in the equilibrium express K_a and K_b either could be used to examine the relationship. We will look at K_a. For the NH₃/NH₄⁺ pair K_a is

\[\rm{K_a ={{[H_3O^+][NH_3]} \over [NH_4^+]} = {[H_3O^+] \times {[NH_3]} \over [NH_4^+]}}\]

K_a has a fixed value. For NH₄⁺ it is 5.56 x 10^-10. What is important is that it is a constant. We are interested in the ratio of the deprotonated form to the protonated form ([NH₃]/[NH₄⁺]. We are considering a solution with a given pH, which means that the [H₃O⁺] is known. Since the product of the [H₃O⁺] and the ratio [NH₃]/[NH₄⁺] is a constant, the larger the [H₃O⁺] the smaller the ratio. This means the [H₃O⁺] increases as the amount of the protonated form increases relative to the deprotonated form. We could have easily determined this from the application of LeChatelier's Principle. Since we know K_a we can determine this relationship quantitatively. Taking the log of both sides of the equilibrium expression yields

\[ \begin{aligned} {\rm log(K_a) = log \left({[H_3O^+] \times {[NH_3] \over [NH_4^+]}} \right) \\ log(K_a) = log([H_3O^+]) + log \left({[NH_3] \over [NH_4^+]} \right) \\ -pK_a = -pH + log \left({[NH_3] \over [NH_4^+]} \right) }\end{aligned}\]

Multiply through by a negative sign (and inverting the logarithm) yields.

\[ \begin{aligned} {\rm pK_a = pH + log \left({[NH_4^+] \over [NH_3]} \right) \\ pK_a - pH = log \left({[NH_4^+] \over [NH_3]} \right) }\end{aligned}\]

Therefore the ratio of the concentration of the protonated form to the deprotonated form depends on the pH compared to the pK_a. A very important condition exists when the pH = pK_a. Then the difference between the two will be zero implying that the ratio of the two species are equal. When the pH = pK_a the solution will contain equal concentrations of both the protonated and deprotonated forms of the compound. What will the ratio be at other pH values? This can be deduced from the formula, but it is easier to simply think about. When the pH < pK_a the solution is "more acidic". The excess protons will protonate the compound and the concentration of the protonated form will be larger than the concentration of the deprotonated form. Similarly if the pH > pK_a the solution is "more basic". This will "pull" the protons off the compound and the concentration of the deprotonated form will be larger than that of the protonated form.

\[ \begin{aligned} {\rm pH = pK_a \;\;\;\; [protonated]=[deprotonated] \\ pH < pK_a \;\;\;\; [protonated]>[deprotonated] \\ pH > pK_a \;\;\;\; [protonated]<[deprotonated] } \end{aligned}\]

This has many implications for chemistry in aqueous solutions (especially for biochemistry). Interactions of molecules and solubility are greatly affected by their charge state. As we protonate or deprotonate compounds their charges, interactions, and solubility will all be changing. The solubility of drug compounds can change dramatically as the pH changes as charged compounds tend to stay in solution (blood) while neutral molecules are absorbed into tissue. This can affect how they are taken up by different regions of the body. Or dramatic difference in drug compounds given orally vs intravenously since stomach acid is dramatically more acidic than blood. Similarly, the structure of proteins can be altered by changing the protonation state of amino-acid residues. As a consequence, the pH in biochemical systems is carefully buffered to be maintained at particular values as fluctuations in pH would have large consequences on protein structure and function.

© 2013 mccord/vandenbout/labrake

scroll to top