For several special cases of rate laws, we can integrate the rate law to yield an equation of the concentration of a particular species as a function of time. It is important to know when such laws apply and in what limits.
In general, these ideas are most useful when the rate of the reactions depends only one one chemical species. This could result from the mechanism of the reaction be true under all conditions for that reaction. Alternatively, we can create situations in which we essentially remove the concentration dependence of one of the reactants by maintaining it at a constant value. Finally, these ideas assume the system is far from equilibrium and that any "backward" reaction (products to reactants) can be ignored.
The goal of an integrated rate law to have an expression for the concentration of the reactants (or products) as a function of time.
By far the most important cases are first order reactions. When a reaction is overall first order with respect to one of the reactants, then the rate of the reaction is simply proportional to the amount of that reactant.
Nuclear decay is an excellent example of a first order process. The rate of decay is simply proportional to the amount of the radioactive isotope. This is due to the fact that any isotope has the same chance of decaying at any given time. Thus the more isotopes there are, the more that can decay (and as time goes on the fewer there are, the fewer that can decay).
Chemical reactions can also be first order. For example the reaction
\[\rm{(CH_3)_3CBr + OH^- \rightarrow (CH_3)_3COH + Br^-}\]
is found to be first order with respect to (CH3)3CBr and does not depend on the concentration of OH-. That means the rate law is
\[\rm{rate = k[(CH_3)_3CBr]}\]
The rate of the reaction can also be written as
\[\rm{rate = {-d[(CH_3)_3CBr] \over dt}}\]
We can combine these two expressions and get a relationship between the rate of change of concentration of the reactant and the concentration of the reactant
\[\rm{{-d[(CH_3)_3CBr] \over dt} = k[(CH_3)_3CBr]}\]
This equation can now be integrated to yield an expression for the concentration of (CH3)3CBr as a function of time.
\[\rm{[(CH_3)_3CBr](t) = [(CH_3)_3CBr]_0 e^{-kt}}\]
That is, the amount of (CH3)3CBr decays away exponentially with time from its initial value at t = 0, [(CH3)3CBr]0. The decay constant, k, is the rate constant.
A generic reaction that is first order in reactant A and zeroth order in all other reactants would yield an integrated rate law of
\[[A](t) = [A]_0e^{-kt}\]
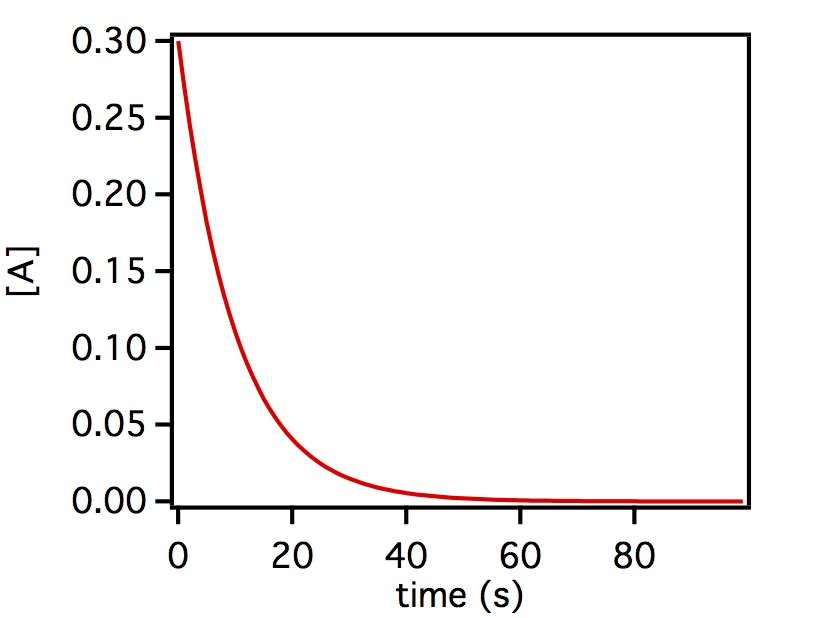
where [A](t) is the concentration of reactant A as a function of time t and [A]0 is the concentration of reactant A at t=0. The concentration decays from this initial value exponentially as shown below.
Above is a plot of a generic first order reaction where the initial concentration of [A] = 0.3 and then decays as a function of time.
It is important to realize that for this to hold the reaction must be first order in A and zeroth order for all other reactants. Additionally, there should be no substantial back reaction. That is, the reaction should really go to completion (or the concentration will deviated from this behavior as the system approaches equilibrium).
First Order Integrated Rate LawSince the concentration is decaying exponentially for a first order reaction, then the natural log of the concentration will decay linearly.
\[[{\rm A}](t) = [{\rm A}]_0e^{-kt}\]
taking the natural log of both sides you get
\[\ln[{\rm A}] = \ln[{\rm A}]_0 - kt\]
This means a plot of the ln[A] vs time will yield a straight line with a slope of -k and and intercept of ln[A]0
On a linear scale the decay will look like this
The natural log plot will look like this
The decay is not a straight line (perfect in this case since it is just simulated data without any noise). The intercept is ln[A]0 = -1.2 (since [A]0 = 0.3, ln(0.3) = -1.2). The slope of the line is -0.1 so k is found to be +0.1 s-1.
The half-life for first order kinetics is quite special since it is a quantity that is related to the rate constant but not the concentration.
This can be easily determined from the integrated rate law. If we write this for a generic first order reactant species, A, the integrated rate law can be written as
\[{[{\rm A}] \over \;[{\rm A}]_0} = e^{-kt}\]
This is now written such that we are looking at the concentration of A relative to the initial concentration. The half-life will be the time at which this ratio is 0.5.
\[0.5 = {[{\rm A}] \over \;[{\rm A}]_0} = e^{-kt_{1/2}}\]
solving this for the half-life, \(t_{1/2}\), we get
\[t_{1/2} = {\ln(0.5) \over -k} = {\ln(2) \over k}\]
First Order Half-LifeIf a reaction is zeroth order overall, it means that the rate of the reaction is independent of all of the concentrations of the reactant. This means that the rate of this reaction will not change as the reaction proceeds. Typically as a reaction progresses, the concentrations of the reactants decreases and the rate decreases. However, for zeroth order reactions, this is not true, the rate is constant in time.
This is because the rate law is
\[\rm{rate = k (constant)}\]
Assuming we are looking at some generic reactant A we can now write
\[\rm{{-d[A] \over dt} = rate = k}\]
this can be easily integrated up to yield
\[\rm{[A] = [A]_0 - kt}\]
The concentration of A is linearly decreasing in time from the initial concentration.
Thus for a zeroth order reaction, a plot of the concentration as a function of time should yield a linear plot.
Zeroth Order Integrated Rate LawA much more limited case, is that of second order reactions. This is highly limited as more 2nd order reactions are the result of bimolecular steps occurring in a reaction. This typically involves a collision between two different molecules. This results in a reaction being 2nd order overall, but one that is first order with respect to two different reactants. In the unusual case that a reaction is second order with respect to a single reactant and zeroth order with respect to all other reactants, we can again come up with an integrated rate law.
\[\rm{rate = k[A]^2 = {-d[A] \over dt}}\]
some calculus follows and you get
\[\rm{{1 \over [A]} = {1 \over [A]_0} + kt}\]
So now a plot of 1/[A] will yield a straight line. The slope is positive since as the concentration is decreasing, one over the concentration is increasing. The slope is simply the rate constant.
Second Order Integrated Rate LawA very important case is that of pseudo-first order kinetics. This is when a reaction is 2nd order overall but is first order with respect to two reactants. This is a very common kinetic scheme.
\[\rm{rate = k[A][B]}\]
where A and B are some generic reactants. Now the kinetics of this reaction can be a bit complicated. The initial rate depends on both A and B and as the reaction proceeds both A and B are changing in concentration and affecting the rate.
When trying to understand this reaction, we can as experimentalists try to set up conditions that simplify things. The easiest way to do this is to try to eliminate the concentration dependence of one of the reactants. We can do this by making the initial concentrations of one of the reactants very very high compared to the other. For example, if we had a reaction
\[\rm{CH_3Br + OH^- \rightarrow CH_3OH + Br^-}\]
For this reaction the rate law is
\[\rm{rate = k[OH^-][CH_3Br]}\]
Imagine we had an initial concentration of CH3Br of 100 μM and and an initial concentration of OH- of 10 mM. Now even if all of the CH3Br has reacted the concentration of OH- will be essentially unchanged. Therefore during the course of the reaction, the concentration of OH- will be essentially constant. This makes the reaction "like a first order reaction", thus the name pseudo-first order.
\[\rm{rate = k[OH^-][CH_3Br]= k(constant)[CH_3Br] = k'[CH_3Br]}\]
Since only concentration of CH3Br would change during the reaction, the rate would only change due to the changes in the CH3Br reaction. Because the reaction is first order with respect to CH3Br, the kinetics would appear to be first order with a new "pseudo-first order rate constant", k'. The value of this rate constant would depend on the value of the overall rate constant, k, and the initial (fixed) concentration of OH-.
© 2013 mccord/vandenbout/labrake