First order reactions

By far the most important cases are first order reactions.  When a reaction is overall first order with respect to one of the reactants, then the rate of the reaction is simply proportional to the amount of that reactant.

Nuclear decay is an excellent example of a first order process.  The rate of decay is simply proportional to the amount of the radioactive isotope.  This is due to the fact that any isotope has the same chance of decaying at any given time.  Thus the more isotopes there are, the more that can decay (and as time goes on the fewer there are, the fewer that can decay).

Chemical reactions can also be first order.  For example the reaction

\[\rm{(CH_3)_3CBr + OH^- \rightarrow (CH_3)_3COH + Br^-}\]

is found to be first order with respect to (CH₃)₃CBr and does not depend on the concentration of OH^-.  That means the rate law is

\[\rm{rate =  k[(CH_3)_3CBr]}\]

The rate of the reaction can also be written as

\[\rm{rate = {-d[(CH_3)_3CBr] \over dt}}\]

We can combine these two expressions and get a relationship between the rate of change of concentration of the reactant and the concentration of the reactant

\[\rm{{-d[(CH_3)_3CBr] \over dt} = k[(CH_3)_3CBr]}\]

This equation can now be integrated to yield an expression for the concentration of (CH₃)₃CBr as a function of time.

\[\rm{[(CH_3)_3CBr](t) = [(CH_3)_3CBr]_0 e^{-kt}}\]

That is, the amount of (CH₃)₃CBr decays away exponentially with time from its initial value at t = 0, [(CH₃)₃CBr]₀.   The decay constant, k, is the rate constant.

A generic reaction that is first order in reactant A and zeroth order in all other reactants would yield an integrated rate law of

\[[A](t) = [A]_0e^{-kt}\]

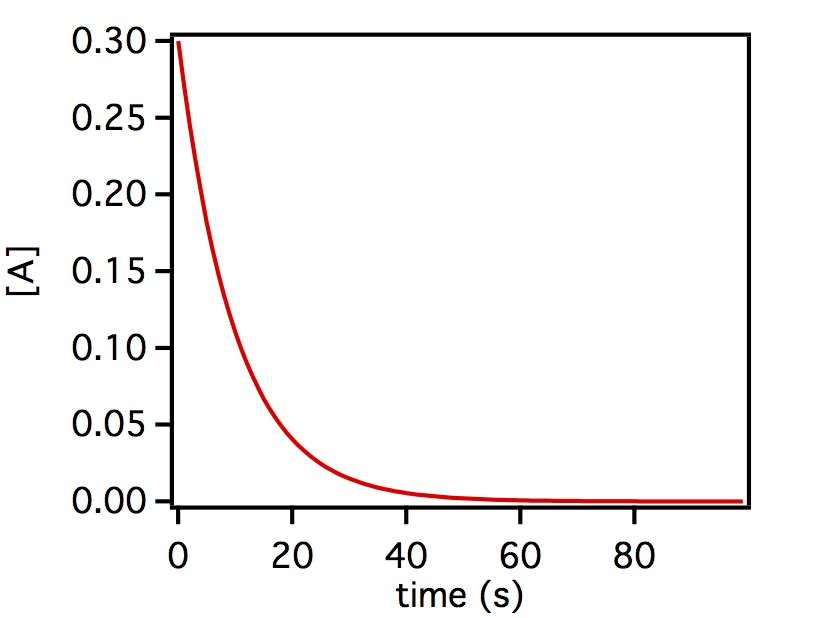
where [A](t) is the concentration of reactant A as a function of time t and [A]₀ is the concentration of reactant A at t=0. The concentration decays from this initial value exponentially as shown below.

Above is a plot of a generic first order reaction where the initial concentration of [A] = 0.3 and then decays as a function of time.

It is important to realize that for this to hold the reaction must be first order in A and zeroth order for all other reactants.  Additionally, there should be no substantial back reaction.  That is, the reaction should really go to completion (or the concentration will deviated from this behavior as the system approaches equilibrium).

First Order Integrated Rate Law